The Wiki for Tale 6 is in read-only mode and is available for archival and reference purposes only. Please visit the current Tale 11 Wiki in the meantime.
If you have any issues with this Wiki, please post in #wiki-editing on Discord or contact Brad in-game.
Difference between revisions of "Guides/Mining/Sand Mining"
| (2 intermediate revisions by the same user not shown) | |||
| Line 84: | Line 84: | ||
|} | |} | ||
| − | == Five Colors (Two Pairs) == | + | == Five Colors (Two Pairs, 3 Single) == |
For a workload distribution of 3 single colors, and then 2 pairs of colors, you can achieve the crumbling of all seven ore stones by following the pattern as laid out below: | For a workload distribution of 3 single colors, and then 2 pairs of colors, you can achieve the crumbling of all seven ore stones by following the pattern as laid out below: | ||
| Line 138: | Line 138: | ||
The same process can be applied to 6- and 7-color fields. Make 6 sets of complementary pairs of workloads, and then one big one to break them all. | The same process can be applied to 6- and 7-color fields. Make 6 sets of complementary pairs of workloads, and then one big one to break them all. | ||
| − | + | == Five Colors - (1 Triple, 4 Single) == | |
| − | |||
| − | == Five Colors - (Triple) == | ||
A triple and five single colours allows us to break 5 stones, which can be done all at once. | A triple and five single colours allows us to break 5 stones, which can be done all at once. | ||
| Line 169: | Line 167: | ||
# A-3, B, C, D, E ''Breaks B, C, D, and E'' | # A-3, B, C, D, E ''Breaks B, C, D, and E'' | ||
|} | |} | ||
| − | |||
| − | |||
== Four Colors (Three Pairs) == | == Four Colors (Three Pairs) == | ||
| Line 215: | Line 211: | ||
# A-2, B-1, C | # A-2, B-1, C | ||
# A-2, B-1, D | # A-2, B-1, D | ||
| + | # A-2, C, D | ||
# A-3, B-1, C | # A-3, B-1, C | ||
# A-3, B-1, D | # A-3, B-1, D | ||
| − | |||
# A-1, B-1, C, D ''Breaks these 4 stones'' | # A-1, B-1, C, D ''Breaks these 4 stones'' | ||
|} | |} | ||
| − | |||
| − | |||
== Four Colors (Quadruple) == | == Four Colors (Quadruple) == | ||
Latest revision as of 14:55, 30 May 2012
Overview
Gems (and coal) are acquired when ore stones in a Mine are broken (by being used in 7 workloads). The size of the gem is random. There is some evidence to suggest that when more ore stones are broken in the same set and/or workload, the gem sizes on average tend to be larger; however, it's still perfectly possible to pull a Huge gem without exploiting this. Sand mines are thus valuable for gem mining, as it's easy to plan in such a way as to break 5+ nodes at once (assuming there are 5 different colors present in the field).
Below are some step-by-step guides for how to get the most gems per workload for various workload configurations.
For the basic mechanics of mining, please refer to the Mining Guide.
Workload Configurations
Seven Colours
For a workload distribution of seven single colors, all stones can be crumbled all at once:
|
Sets
|
Six Colors (One Pair)
For a workload distribution of 5 single colors, and then 1 pairs of colors, you can achieve the crumbling of all seven ore stones by following the pattern as laid out below:
|
Sets
|
Six Colors (Alternate)
It is also possible to form a group of 6 that breaks all at once, if desired.
|
Sets
|
Five Colors (Two Pairs, 3 Single)
For a workload distribution of 3 single colors, and then 2 pairs of colors, you can achieve the crumbling of all seven ore stones by following the pattern as laid out below:
|
Sets
|
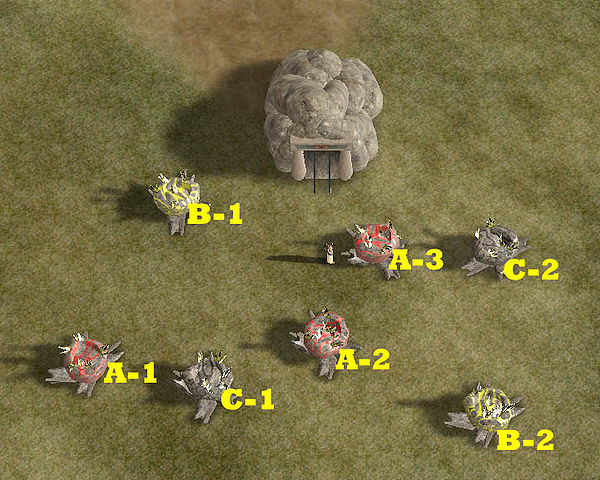
Five Colors - Alternate Method
Using the theory that gem size is tied to the number of stones broken in a single workload (as opposed to the number of total stones broken in a field), the above can be simplified to do a little less work and still get the big break that will be producing most of the big gems.
Refer to the above image.
First, choose one of the duplicate-colored stones to ignore completely. Here we'll choose B-2. Then pick one of the stones in the remaining pair (say, A-1) to be your starter stone. Make any valid 3-stone workload starting with your starter stone, and immediately follow that with the workload consisting of the remaining 3 stones. There are exactly 6 sets of these pairs of workloads. Do them all.
| A-1, C, D | A-2, B-1, E |
| A-1, C, B-1 | A-2, D, E |
| A-1, C, E | A-2, D, B-1 |
| A-1, D, B-1 | A-2, C, E |
| A-1, D, E | A-2, C, B-1 |
| A-1, B-1, E | A-2, C, D |
And finally choose a 5-stone workload (either A-1 or A-2 in addition to B-1, C, D, and E).
The same process can be applied to 6- and 7-color fields. Make 6 sets of complementary pairs of workloads, and then one big one to break them all.
Five Colors - (1 Triple, 4 Single)
A triple and five single colours allows us to break 5 stones, which can be done all at once.
|
Sets
Alternatively, you can replace the last step with these two steps, for more broken stones but fewer at the same time:
|
Four Colors (Three Pairs)
With three pairs of colours all stones can be broken, in a group of 3 and a group of 4.
|
Sets
|
Four Colors (Triple and Pair)
With a triple and a pair of colours it is possible to break 4 stones, which can be done all at once.
|
Sets
|
Four Colors (Quadruple)
With a quadruple of a colour it is possible to break 6 stones, in two groups of 3.
|
Sets
|
Three Colors (Triple and Two Pairs)
When we have a triple and two pairs of colours, no stone can be worked seven times. Just do a few workloads to speed up resetting the mine.
|
Sets None. |
Three Colors (Quadruple and Pair)
When there is a quadruple and a pair of colours, only the single stone can be broken.
|
Sets
|
Three Colors (Quintuple)
When there is a quintuple, five stones can be broken.
|
Sets
|
Three Colors (Two Triple)
It's possible to break one stone.